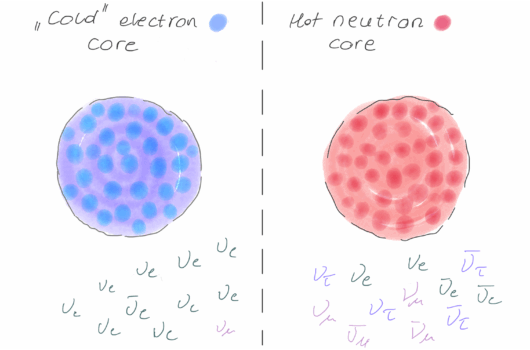
I study the flavor evolution of a dense neutrino gas by considering vacuum contributions, matter effects, and neutrino self-interactions. Assuming a system of two flavors in a uniform matter background, the time evolution of the many-body system in discretized momentum space is computed. The multiangle neutrino-neutrino interactions are treated exactly and compared to both the single-angle approximation and mean-field calculations. The monoenergetic two-neutrino beam scenario is solved analytically. I proceed to solve flavor oscillations for monoenergetic cubic lattices and quadratic lattices of two energy levels. In addition I study various configurations of 12, 16, and 20 neutrinos. I find that when all neutrinos are initially of the same flavor, all methods agree. When both flavors are present, I find collective oscillations and flavor equilibration develop in the many-body treatment but not in the mean-field method. This difference persists in dense matter with tiny mixing angle and it can be ascribed to non-negligible flavor polarization correlations being present. Entanglement entropy is significant in all such cases. The relevance for supernovae or neutron stars mergers is contingent upon the value of the normalization volume V and the large N dependence of the timescale associated with oscillations. In future work, I intend study this dependence using larger lattices and also include antineutrinos.